
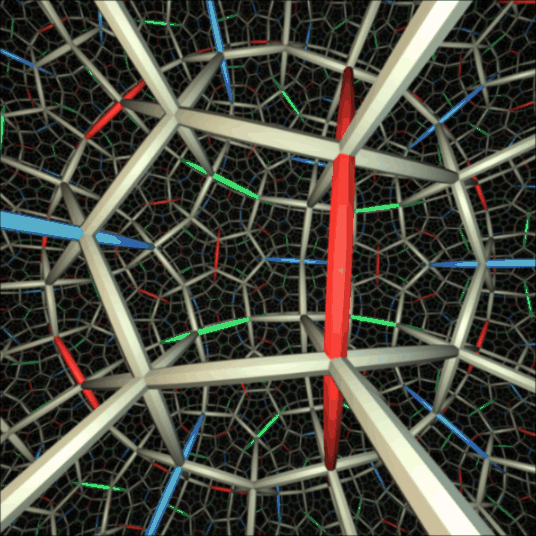
In non-Euclidean geometry, angles and distances behave differently from what our Euclidean intuition leads us to expect. This figure shows how a hyperbolic space can be tesselated by right-angled dodecahedra.
Non-Euclidean geometry is the mathematical framework for the description of our physical time-space in general relativity; concepts of non-Euclidean geometry are related to many other mathematical fields. An example: the complement of the knot we saw earlier, that is what remains from space after you "take away" the knot, has the structure of a hyperbolic space of constant (negative) curvature.
Figure credit: C. Gunn.
URL for this and other geometry pictures: http://www.geom.umn.edu/docs/research/ieee94/fig